Rigid Body Transformations
Notations
- represents the orientation of frame with respect to frame .
- represents the translation of frame with respect to frame .
- represents the homogeneous transformations of frame with respect to frame .
Where, in 3d, is a 3 3 matrix, is a vector.
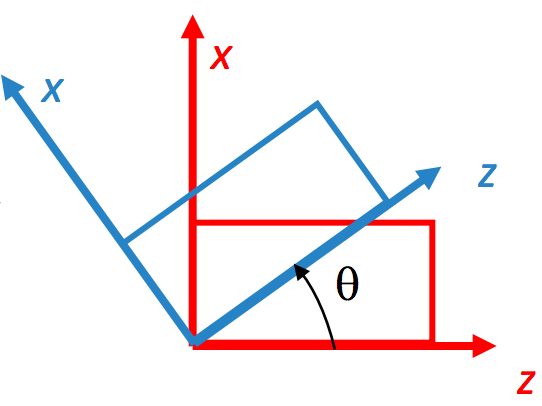
- represent rotations about the axis (right-hand rule) by angle
Composite Transformations
- Post-multiply successive transformations about intermediate frames
- Pre-multiply successive transformations about fixed frames
Composition of homogeneous transformations follows the rules of rotation matrices.
Inverse Transformations
- Rotations
- Homogeneous transformations
Skew Symmetric Matrices
is the skew symmetrix matrix.
For any skew symmetrix matrix .
2D Rotation
The rotation in 2d can be viewed as the complex number
Rodrigues’ Formula
Rodrigues’ formula gives us an decomposition of the rotation matrix into axis and angle.
Quaternions
Quatornion is a 4 dimensional representation of the rotation matrix. The basis are . The quatornion is a generalization of complex number.
- Unit Quatornion Properties
-
Relation to angle-axis
-
Not unique
-
Commutativity
-
Conjugate
-
Inverse
-
Norm
-
Multiplication
-
Operation on a vector ( is representing rotation matrix )
-